AUCTORES
Globalize your Research
Research Article | DOI: https://doi.org/10.31579/2637-8914/267
1 Víctor A. Reale, Centro de Investigación y Desarrollo en Criotecnología de Alimentos (CIDCA). Facultad de Ciencias Exactas, National University of La Plata, Argentina.
2 Demarchi, S.M., Centro de Investigación y Desarrollo en Criotecnología de Alimentos (CIDCA). Facultad de Ciencias Exactas, National University of La Plata, Argentina.
3 R. Martin Torrez Irigoyen, Centro de Investigación y Desarrollo en Criotecnología de Alimentos (CIDCA). Facultad de Ciencias Exactas, National University of La Plata, Argentina.
*Corresponding Author: R. Martin Torrez Irigoyen, National University of La Plata, 47 and 116 Streets (1900) - La Plata, Buenos Aires, Argentina.
Citation: Víctor A. Reale, Demarchi, S.M., R. Martin Torrez Irigoyen, (2024), Modeling of Freeze-drying Kinetics for Apple, Banana, and Strawberry, J. Nutrition and Food Processing, 7(13); DOI:10.31579/2637-8914/267
Copyright: © 2024, R. Martin Torrez Irigoyen. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Received: 27 September 2024 | Accepted: 14 October 2024 | Published: 30 October 2024
Keywords: freeze-drying; mathematical model; sublimation; desorption; fruits
Fresh apple, banana, and strawberry slices were frozen at -20°C and freeze-dried using a shelf temperature of 40°C. Theoretical expressions were developed to predict vapor transfer kinetics during both sublimation and desorption periods. For sublimation, a model that accounts for the increasing dried layer thickness was employed to predict the sublimation rate as a function of time. This model significantly improves upon the time equation found in literature without adding substantial complexity. For desorption period, an analytical solution of the unsteady-state diffusion equation was applied. Permeabilities were determined for the sublimation drying model at an absolute pressure of approximately 30 Pa. However, the relevant kinetic coefficient combines permeability and the mass of ice to sublime relative to the dry matter (sublimation kinetic coefficient). In the desorption drying model, diffusion coefficients of vapor in the dried layer were on the order of 1×10-9 m²/s for pressures around 3-5 Pa. In both periods, the agreement between predicted and experimental values was highly satisfactory. A minimum freeze-drying time of 12, 6.8, and 8.7 hours, respectively, was calculated for apple, banana, and strawberry, considering a final moisture content of 4% w/w. Normalized drying curves revealed a faster sublimation rate for banana, intermediate for strawberry, and slowest for apple. Conversely, desorption curves showed a faster desorption rate for apple, intermediate for banana, and slower for strawberry. In each period, the order of the relevant kinetic coefficients corresponded to the Arrangement of the experimental curves.
b Dried layer permeability to the vapor flux, [kg water (m Pa s)-1]
C2m Parameter defined in Equation (15), [m Pa kg water-1]
D Water vapor diffusion coefficient in the dried layer, [m2 s-1]
Fice Frozen water fraction in the sample, [kg ice kg initial water-1]
G Sublimation rate per unit area in the primary drying period [kg water m-2 s-1]
kg Mass transfer coefficient between sample top surface and condenser, [kg water (m2 Pa s)-1]
ks Sublimation kinetic coefficient, [s-1]
L Thickness of material, [m]
m Moisture content (average in sample) at time t, [kg water kg dry matter-1]
m0 Initial moisture content, [kg water kg dry matter-1]
me Final moisture content for the primary drying period, [kg water kg dry matter-1]
ml Local moisture content at time t, in the desorption period, [kg water kg dry matter-1]
meq Equilibrium moisture content, [kg water kg dry matter-1]
mdd Dimensionless mean moisture content
Piw Vapor pressure of ice in the sublimation front, [Pa]
Psw Vapor pressure at the surface of the dried layer, [Pa]
Paw Vapor pressure at the condenser surface, [Pa]
Pw Pressure at the solid-vapor interface, [Pa]
Tlp Shelf temperature, [K]
Ti Temperature of ice in the sublimation front, [K]
Ts Dried layer surface Temperature [K]
Taf Air temperature in the batch freezer [°C]
Tf Initial freezing temperature of product, [°C]
t Time, [s]
tsp Duration of the sublimation period [s]
tdp Duration of the desorption period [s]
tfd Duration of the total freeze-drying process [s]
xd Dried layer thickness, [m]
Y Fraction of residual ice content at time t defined in Equation (8), [dimensionless]
Greek symbols
ρd Dry matter density, [kg dry matter m-3]
ρf Frozen food density, [kg m-3]
Freeze-drying is a novel process that removes water from a previously frozen product through sublimation during the first period and desorption during the secondary stage [1]. Freeze-dried products are recognized for their superior quality among dehydrated foods, preserving bioactive compounds and maintaining structural integrity, thereby preventing shrinkage. Although more expensive due to longer drying times and higher energy consumption, freeze-drying is adequate for high-value products like pharmaceuticals and certain foods, such as strawberries, carrots, red pepper, mushrooms, apples, and bananas [2, 3, 4, 5]. Despite the increased investment and processing costs, the growing consumer demand for convenience and quality is conducting the production of more freeze-dried foods. However, further research, particularly in freeze-drying kinetics, is needed to develop accurate mathematical models that can enhance our understanding of the process, estimating process time and other design parameters [3]. While relatively simple models exist for estimating sublimation time and the variation in moisture content, most assume heat conduction from the bottom and vapor diffusion to the top [6, 7].
Some authors, as James and Datta (2002) [8] developed a drying model for carrot slices, focusing on the sublimation stage. Their model neglected surface-to-condenser mass transfer, concluding that the process was mass transfer-controlled. Further studies carried on in mushrooms and red pepper revealed faster drying for red pepper, likely due to differences in structure or composition [8]. Other drying models incorporate heat transfer from both, top and bottom surfaces, requiring different mathematical treatments. Authors as El-Maghlany et al. (2019) [9] proposed a more complex model for the sublimation stage, considering pore-based transfer mechanisms. However, this study was limited to the primary stage. For other hand, Sadikoglu and Liapis (1997) [10] developed models for both primary and secondary periods in bulk solution freeze-drying, considering conduction and radiation heat transfer and upward vapor water diffusion. While literature usually focuses on complex models, some intermediate-complexity models that enhance the sublimation time model developed by Karel and Lund (2003) [11] have received less attention. However, this model, limited to zero ice content, fails to capture the influence of the growing dried layer during the primary drying stage. In light of the literature, predicting water content as a function of time during this phase has been infrequently modeled. Furthermore, the secondary drying stage, involving desorption and diffusion through the dried layer, consumes a significant portion of the total drying time despite representing a small fraction of the initial water content.
2.1. Conditioning of raw materials
Slices of peeled apple (Red Delicious), banana (Musa Paradisiaca), and strawberry (Fragaria x Ananassa), were acquired from a local market. The fruits were cut in slices from 0.01 m in thick with a sharp knife. Samples were placed in 0.3 m diameter trays, in turn covered with food grade PVC film and introduced in a freezer at -20 °C for 24 h. The tray cover avoided some dehydration that might occur during freezing and while the sample was moved from the freezer to the freeze-dryer chamber.
2.2. Equipment description
A freeze dryer model L-A-B4-C was used (RIFICOR, Argentina, http://www.rificor.com.ar/). The equipment consists of a cylindrical vacuum chamber made of transparent acrylic covering a stainless-steel framework holding four disc-shaped shelves spaced 0.07 m. The shelves have built-in heating elements and a Pt-100 temperature sensor connected to a temperature automatic control up to 50 °C. Stainless steel trays 1 mm thick, 0.3 m diameter, with a lateral wall 0.02 m high, were placed with the samples. The equipment is fitted with a Pt-100 product temperature sensor, covered by a metallic case, and connected to a digital display. The chamber pressure was measured with a Pirani gauge, and the results continuously shown in a digital display. The equipment can be observed in Figures 1 and 2.
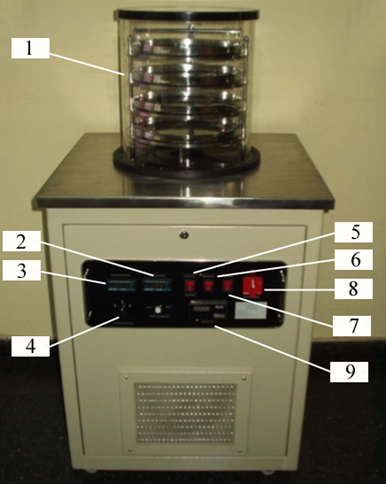
Figure Legend 1: Rificor Freeze Dryer model L-A-B4-C. 1. Vacuum chamber 2. Shelf temperature control; 3. Display showing either shelf, product, or condenser temperature; 4. Switch to select the temperature being displayed 5. Switch that starts condenser and its temperature measurement 6. Switch for starting vacuum pump and pressure gauge; 7. Switch to start heating to shelves 8. Main switch 9. Absolute pressure gauge.

Figure Legend 2: Vacuum chamber of the Rificor L-A-B4-C Freeze dryer. 1. Tray; 2. Transparent vacuum chamber; 3. Temperature-controlled shelf. 4. Product temperature sensor; 5. Framework supporting the structure of the shelves under high vacuum.
2.3. Freeze-drying process
One tray with the frozen fruit was removed from the freezer, uncovered and placed in the freeze-dryer as the condenser temperature reached -48 °C. The cylindrical acrylic cover was put in place, and the vacuum pump was started. Chamber pressure was closely monitored and as soon as a value of 30 Pa was reached, shelf heating was switched on to set a target value of 40 °C. This last action was considered zero time for freeze-drying, i.e. To determine the experimental curve of moisture content as a function of time, triplicate experiments were carried on between 1.5 and 24 h. Moisture content for fresh and freeze-dried fruits were determined in an Arcano (China) vacuum oven connected to a Vacuubrand PC 500 Series – CVC 3000 (Germany) diaphragm vacuum pump for 6 h at 70 °C, following the AOAC 934.06 method [12].
3.1. Theoretical considerations
3.1.1 Sublimation model
The food slices (assumed a plane sheet) was subjected to heat transfer from both above and below. Conduction from the lower shelf and radiation from the upper shelf contributed to heating. This was observed during preliminary freeze-drying experiences, where a dried layer formed symmetrically above and below the frozen zone. Consequently, vapor was assumed to diffuse through both surfaces and the characteristic vapor migration became half the initial sample thickness. Therefore, symmetrical transfer of heat and mass was considered throughout the process. The scheme transfer phenomena is presented in Figure 3.
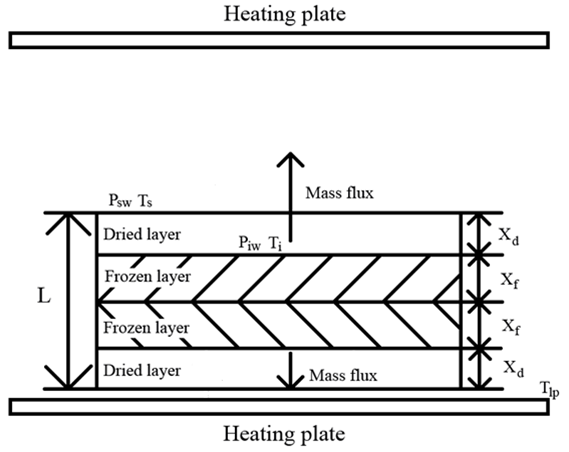
Figure Legend 3: Schematic of freeze-drying in the sample during the sublimation period.
The sublimation rate per unit area G, depends on the mass transfer as shown by Equation (1)

Where xd is the dried layer thickness; Piw, the vapor pressure in the sublimation front and Psw the vapor pressure at the surface of the dried layer. Symbol b is the dried layer permeability to water vapor. In addition, the vapor transfer between the top surface and the condenser can be represented by:

The symbol kg stands for the mass transfer coefficient between the dried layer top surface and the condenser, which depends on equipment design and operating variables. The symbol, Paw is the vapor pressure at the condenser temperature of -48 °C.
Ice temperature measured at the sublimation front were of -19, -18 and -22 °C for apple, banana, and strawberry, respectively. The vapor pressure of ice in the sublimation front were calculated by the following correlation [13]:
Pw=exp31.96-6270.36T+273.15-0.461 ln(T+273.15)

Using Equation (3), values of Piw resulted 113.9 for apple, 125.2 in banana and 85.3 Pa in strawberry, being Paw of 5.0 Pa. As Equation (1) and (2) are different expressions for the same vapor flux, both can be equated as follows

Although Piw and Paw keep constant in the primary drying period, Psw becomes a function of the dry layer thickness xd. By solving Equation (4) for Psw we achieve the expression:

This equation includes two parameters: b and kg. By replacing Equation (5) into Equation (2), and rearranging, the sublimation rate can be expressed in terms of the following flux equation:

Equation (6) predicts a time-varying vapor rate per unit area which is part of the transient macroscopic mass balance
| Rate of accumulation of vapor inside the sample | = | Transfer rate through the dried layer out of the sample and towards the condenser |
The accumulation rate per unit area can be expressed as follows

Where ρd is the density of the dry material, being t the instantaneous time. The negative sign must be written as dm/dt is inherently negative in dehydration. Where m stands for the moisture content, decimal dry basis at time t. The model would be more general by normalizing the ratio of frozen water remaining (m – me) relative to the initial frozen water (m0 – me) given by the expression

Most models involving a dependent dimensionless variable would tend asymptotically to a limiting value, though that behavior is not expected for Y in the sublimation period, as me is not an equilibrium moisture content, but the maximum unfrozen water content for a fruit freeze dried at the prevailing operating conditions. Therefore, experimental data should present a change in the drying mechanism (approximately for a time where m≈me) from ice sublimation to water desorption.
By assuming uniform internal moisture distribution (a reasonable approximation in a sublimation front), the ratio of frozen water removed by sublimation relative to the initial frozen water content available for sublimation is 1–Y, which can be considered equivalent to the ratio of the dried layer thickness to the initial half thickness of the sample. This is represented by the following expression

Being L the sample thickness. Now, by deriving Equation (8) with respect to time, a relationship is obtained between m and Y

Replacing Equation (10) into Equation (7) and rearranging, the accumulation term becomes

The dry matter density is calculated from the value of the frozen food by assuming constant sample volume of during the sublimation period, as shown in the equation below

Where ρf is the frozen food density. Now, by combining Equation (6) and (11)

Now, by solving for xd in Equation (9), replacing it in Equation (13), multiplying both sides of the equal sign by 2/L and rearranging, the following expression is reached:

To simplify the writing, some variables keeping constant during sublimation were grouped and termed C2m:

Multiplying both sides of Equation (15) by the dried layer permeability b

By integrating from Y=1 to a generic Y in the left member, and from 0 to t in the right member, we have

Multiplying both members by (-2) and grouping part of the results in a binomial, an intermediate expression is found

with the purpose of grouping variables again in a binomial, the term (2b / (kg L))2 is added at both sides of the equal sign to allow for the following equation

By solving for Y, the first version of the model for the sublimation period is achieved

To normalize experimental moisture contents (Equation (8)) for fitting Equation (20) to them, the moisture content at the end of the sublimation period (me) is calculated from the fraction of unfrozen water in the previous freezing stage at -20 °C. This criterion is considered well-founded and original, and me does not only determine the endpoint of sublimation but also the starting point for the secondary period. To estimate the frozen water fraction, a correlation by Fikiin (1998) [14], accurate for fruits, was employed:

Where Fice is the fraction of frozen water in the sample, being Taf the air temperature in the freezer and Tf the initial freezing temperature. Therefore, the fraction of unfrozen water 1 - Fice, can be used to calculate a delimiting moisture content between the primary and secondary drying periods

3.1.1.1 Fitting of the sublimation model
Parameters and properties utilized here are listed in Table 1 [15, 16].
| Apple | Banana | Strawberry | |
| ρf (kg m-3) | 787 | 863 | 882 |
| ρd (kg m-3) | 116.79 | 214.73 | 88.02 |
| m0 (kg water kg dry matter-1) | 5.738 | 3.019 | 9.021 |
| me (kg water kg dry matter-1) | 0.625 | 0.353 | 0.981 |
| Tf (°C)b | –1.45 | –3.88 | –1.39 |
| Tlp(°C) | 40 | 40 | 40 |
| L (m) | 0.01 | 0.01 | 0.01 |
| Taf (°C) | –20 | –20 | –20 |
| Tiw (°C) | –19 | –18 | –22 |
| Piw (Pa) | 113.9 | 125.3 | 85.3 |
| Paw (Pa) | 5.0 | 5.0 | 5.0 |
Table 1: Properties and operating conditions utilized for the sublimation drying model (Eq. (21)).
Experimental moisture contents and time were selected for the primary drying period, and moisture contents converted into the dimensionless variable Y as indicated by Equation (8), while Equation (20) was programmed in a user-defined MATLAB function. Equations and Figures were programmed and plotted in MATLAB 7.5.
Initial estimates for b and kg were provided for the built-in function nlinfit to which the experimental data of Y vs t were supplied. The program thus written was able to determine the optimizing parameters b and kg by nonlinear least squares, and the regression coefficient of determination, r2. Fitting parameters for each fruit in this sublimation period were presented in Table 2.
| Apple | Banana | Strawberry | |
Ice fraction during freezing (kg ice kg initial water-1) | 0.8911 | 0.8831 | 0.8912 |
| Duration of the sublimation period (h) | 8.5 ± 0.26 | 4.0 ± 0.44 | 5.4 ± 0.58 |
Permeability b (kg water (m Pa s)-1) | 2.242×10-9 ± 5.99×10-11a | 4.197×10-9 ± 4.43×10-10 b | 5.644×10-9 ± 5.18×10-10c |
Convective mass transfer coefficient kg (kg water (m2 Pa s)-1) | 1.728×10-6 ± 8.31×10-7d | 72.087 ± 18.936e | 1.334×10-5 ± 6.17×10-6f |
Coefficient of determination r2 | 0.9799 | 0.9910 | 0.9532 |
a,b,c Average ± Standard Deviation (n=3) with different superscript letters on the same row are significantly different (α<0>
d,e,f Average ± Standard Deviation (n=3) with different superscript letters on the same row are significantly different (α<0>
Table 2: Preliminary parameter estimation for the sublimation model.
In Equation (20) two parameters of considerably different order of magnitude has been obtained, and, although Table 2 show that the expression provided accurate predictions, one must consider that the regression algorithm optimizes the parameters regardless of their physical meaning and in this sense, large variations were observed for kg which makes it unreliable and a low variation for parameter b. Hence, by neglecting the external resistance to mass transfer, Equation (20) becomes
 (23)
(23)
Provided Equation (23) can maintain accurate predictions, more meaningful values of the dried layer permeability for each fruit might be determined. Fitting results of Equation (23) are presented in Table 3. A small loss of accuracy can be noticed only in apple but not in banana nor strawberry.
| Apple | Banana | Strawberry | |
Ice fraction during freezing (kg ice kg initial water-1) | 0.891 | 0.883 | 0.891 |
Duration of the sublimation period (h) | 8.5 ± 0.26 | 3.9 ± 0.34 | 5.5 ± 0.58 |
Permeability b (kg water (m Pa s)-1) | 2.433×10-9 ± 6.02×10-11a | 4.248×10-9 ± 3.61×10-10b | 5.538×10-9± 5.17×10-10c |
Sublimation kinetic coefficient ks (s-1) | 1.309×10-4 ± 4.12×10-6d | 2.846×10-4 ± 2.46×10-5e | 2.019×10-4 ± 2.19×10-5f |
Coefficient of determination r2 | 0.9464 | 0.991 | 0.9502 |
a,b,c Average ± Standard Deviation (n=3) with different superscript letters on the same row are significantly different (α<0>
d,e,f Average ± Standard Deviation (n=3) with different superscript letters on the same row are significantly different (α<0>
Table 3: Results for the primary drying period.
Now that the model has been simplified C2m can be expressed in its form of Equation (15) not to conceal the factors affecting the curve
 (24)
(24)
While the dried layer permeabilities, a kinetic parameter, are ordered from highest to lowest as strawberry > banana > apple, the plots of dimensionless Y vs dimensional t show the following order in drying rate: banana (fastest) > strawberry > apple (lowest). This behavior is probably due to the curve is not explained solely by b, there are two consecutive steps: (1) sublimation of ice and (2) migration through the pores. Permeabilities explain migration but not sublimation, which can be described particularly by m0-me, i.e, the mass of ice sublimed relative to the dry matter. Thus, a parameter called sublimation kinetic coefficient ks is defined:

Which leads to the final form of the model for the sublimation period:
 (26)
(26)
Table 3 shows the values calculated for ks. In this case, the ordering of this kinetic coefficient is coincident with the order of sublimation rates of curves presented in Figure 4. Banana is less porous than strawberry though its mass of ice to sublime per kg of dry matter is also lower.
The values of b determined here for apple, banana, and strawberry are comparable to the 3.5×10-8 kg water (m Pa s)-1 found by Quast and Karel (1968) [17] in freeze-dried coffee. Values were also in the order of the 1.5×10-8 kg water (m Pa s)-1 published by Sandall, King and Wilke (1968) [18] for turkey breast and to 1.8×10-8 kg water (m Pa s)-1 determined by Hill (1967) [19] for beef.
Experimental data of Y vs t and predictions of the model in any of its equivalent forms (Equation (23), (24) or (26)), with the fitting parameter b for the sublimation period are plotted in Figure 4.
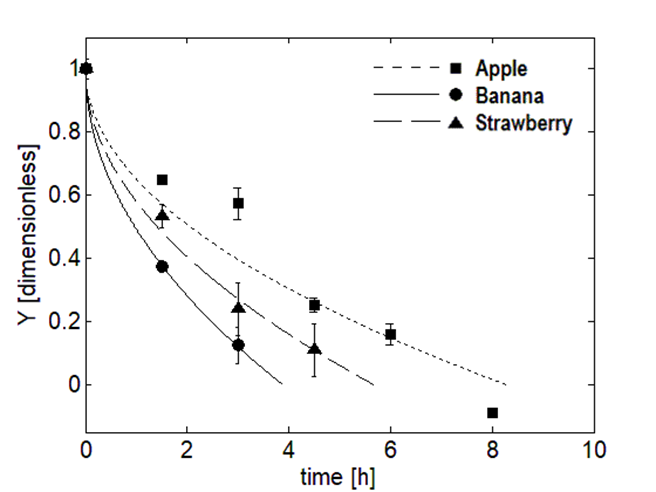
Figure Legend 4: Experimental and predicted (Eq. 26) normalized moisture content ((m-me)/(m0-me)) as a function of time during primary drying of apples, bananas, and strawberries. Error bars represent standard deviations of the data.
In Figure 4, the calculated values closely match the experimental data, demonstrating substantial accuracy for this difficult experimental system. The sublimation rate gradually decreases (in absolute value) due to the growing dried layer thickness during sublimation. This behavior was not clearly explained in the literature, which often compares the sublimation period with the convective drying of high-moisture foods, despite the latter provides a linear behavior [3].
3.1.2 Desorption model
The remaining unfrozen moisture is bound to the food matrix and has a lower vapor pressure than pure liquid at the same temperature. This “bound moisture” concept is often used but can be ambiguous. In this study, we prefer the term “adsorbed water”. For secondary drying, adsorbed water must be desorbed and diffuse as vapor through the dried layer, exiting the sample towards the condenser. To model this process, an unsteady-state mass balance was proposed, based on Fick's law of diffusion [20]:

Where ml stands for the local moisture content in the dried layer, now occupying the entire thickness of the sample, being D the effective vapor diffusion coefficient. The initial and boundary conditions were:



The time t is counted now from the start of the desorption period. The value of meq is the equilibrium moisture content at the operating conditions prevailing in the experiments, [kg water kg dry matter-1]. In the desorption period, and, because of the high vacuum conditions, this equilibrium value was assumed zero.
Considering no shrinkage and constant volume (constant diffusion coefficient), Equation (27) together with the initial and boundary conditions Equation (28) to (30), can be integrated over the half volume of the sample. These assumptions are substantially met during desorption in a freeze-drying process. The analytical series solution is:


Where mdd is the dimensionless mean moisture content. As mentioned above, the starting moisture content in the desorption period (me) coincides with the final moisture in the sublimation stage.
This combined equation was solved for the average moisture content, m, to fit the experimental data of the desorption period using a method previously described for the sublimation period, but now optimize parameter D. The moisture content-time data from the desorption period were not used in fitting the sublimation model. The moisture content corresponding to the unfrozen water fraction, me, was considered a pseudo-experimental point. For m=me, zero time was assumed for the start of secondary drying. The duration of the primary period was previously calculated by the sublimation model as the time required for moisture content to decrease from m0 to me. Therefore, the times used during the secondary period in the fitting were the cumulative time minus the sublimation time. This is possible because the secondary drying period is assumed to begin with no moisture content gradients throughout the thickness.
Equation (31) and (32) are written in a user-defined function file. The program module allows a variable number of terms to be employed, and the sum in Equation (31) is terminated for each time as the last term falls below 1.0×10-5. With this adaptive programming, a lower number of terms are used towards the end of each fitting exercise. The optimized value of D and the goodness of fit parameters are presented in Table 4.
| Apple | Banana | Strawberry | |
Diffusion coefficient (m2 s-1) | 1.628×10-9 ± 2.554×10-11a | 1.977×10-9 ± 1.055×10-9a | 2.285×10-9 ± 2.213×10-9a |
Coefficient of determination r2 | 0.9999 | 0.9790 | 0.9762 |
Duration of the desorption stage (h) | 4.3 ± 0.10 | 3.2 ± 1.35 | 3.3 ± 1.92 |
Duration of the freeze drying process (h) | 12.8 ± 0.36 | 7.1 ± 1.11 | 8.9 ± 1.34 |
a Average ± Standard Deviation (n=3) with different superscript letters on the same row are not significantly different (α<0>
Table 4: Results of the desorption model fitting to the experimental data.
The coefficients of determination demonstrate that the predictions for the secondary period were generally satisfactory, being highly accurate in apple, accurate in banana and still very good in strawberry. All calculations required only a few seconds of computing time, indicating the model's potential usefulness in control algorithms. According to the glass transition theory, a critical moisture content must be defined to approach the glassy state of a dry solid, ensuring long-term food stability. For this reason, a final moisture content of 4% w/w or 0.0416 kg water per kg dry matter was used. This value was utilized to calculate the secondary freeze-drying time. Several studies on glass transition phenomena in freeze-dried fruits have suggested a similar final moisture content as suitable for preserving freeze-dried fruits at ambient temperature [21, 22, 23]. The total freeze-drying time is shown in Equation (33)

where tdp is the duration of desorption period, tfd, the length of the total freeze-drying process, while tsp stands for the duration of the sublimation period, all times being in [s].
Predictions of the model were in fair agreement with experimental mdd as a function of time as observed in Figure 5 for the three fruits. Times were converted to h in the graph for easier visualization.
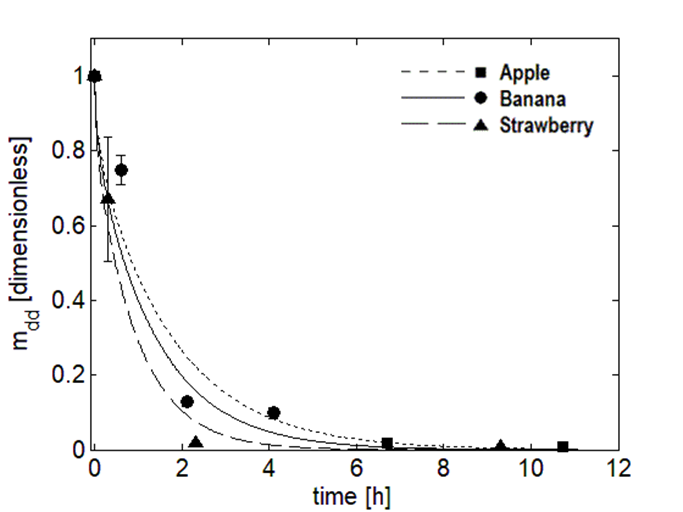
Figure Legend 5: Dimensionless moisture content as a function of desorption time: apple (slowest drying curve), banana (medium drying curve), and strawberry (fastest drying curve). Values predicted by Equations 31 and 32 are compared to experimental data, with standard deviations plotted as error bars.
As shown in Figure 4, the curve order aligns with the order of the sublimation kinetic coefficient, ks. This is because, as previously discussed, ks depends not only on permeability, b, but also on the relative amount of ice being sublimed compared to the dry matter. In contrast, in Figure 5, the curve order follows the same mode as the vapor diffusion coefficients because, during the latter period, the only significant mass transfer parameter is D, which relates to the structure and its porosity. As moisture content at the end of the process is considerably low and more susceptible to errors compared to values obtained during the sublimation period triplicate experiments are especially valuable in the desorption period, particularly towards its end.
The diffusion coefficient determined here for apples was slightly higher than that reported by Saravacos (1967) [24] for the same freeze-dried fruit, 0.7×10-9 m2 s-1. This difference can be attributed to the higher shelf temperature of 40 °C used in this study compared to the 30 °C used by this authors. In contrast, the diffusivity for banana slices air-dried at 38 °C, 2.1×10-10 m2 s-1, was much lower than in this work. Atmospheric pressure generally tends to increase the diffusion coefficient, but the collapsed structure of an air-dried fruit significantly reduces this parameter [25]. No diffusion coefficients during freeze-drying studies were found for strawberry. Interestingly, when comparing Tables 3 and 4, readers will notice that the order of permeabilities during the sublimation period coincides with the order of diffusion coefficients during the desorption stage (apple < banana>b and D, which are related to the movement of water vapor through the porous structure of the dried layer.
Table 3 and 4 present the most representative parameters for the primary and secondary drying periods: permeability and the diffusion coefficient, respectively. A statistical analysis of variance (ANOVA) was conducted (α=0.05) to determine if the differences between the obtained parameters were significant. Regarding the permeability, the results indicated significant differences among the values for each fruit. This can be attributed to differences in their structure, chemical composition, and initial moisture content. These factors influence the dried layer thickness and the amount of ice per kg of dry matter, directly affecting the value of b for each fruit. On the other hand, no significant differences were found between the diffusion coefficients. This may be associated with the complete sublimation of ice during this period, allowing the remaining water to move through the pores of the dry matter. At such low moisture contents, it is reasonable to assume that the diffusion coefficient would not exhibit significant variations. Similar conclusions were mentioned by Chen et al. (2023) [26] who modeled the mass and energy transfer during kiwi freeze-drying.
Predictions of both models adapted for the moisture content dry basis normalized by the initial moisture content as a function of time, together with the experimental data for the two periods (Equations (8), (26), (31) and (32)) are plotted in Figure 6.
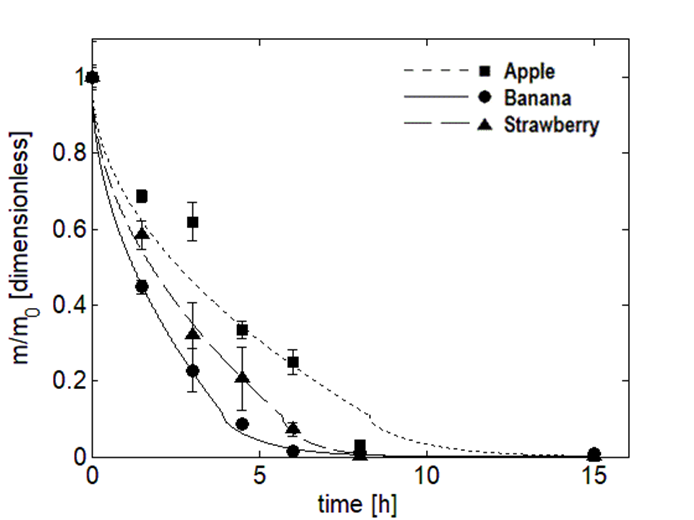
Figure Legend 6: Normalized moisture content as a function of time during the complete freeze-drying process: primary and secondary drying models. Apples, bananas, and strawberries. Values were predicted using Eqs. 26, 31, and 32. Standard deviations for experimental values are represented by error bars.
Figure 6 demonstrates that the predictions closely follow the experimental behavior. The transition between the predictions of the primary and secondary period models is marked by a change of slope. Although continuity of moisture content was ensured between the models, the derivatives were not continuous due to the different drying mechanisms in the two periods. Finally, Figure 7 presents some images of each fruit before and after the freeze-drying process. As shown, there is minimal difference between the initial and final appearance of the fruits, highlighting one of the most appealing aspects of this drying method [27].
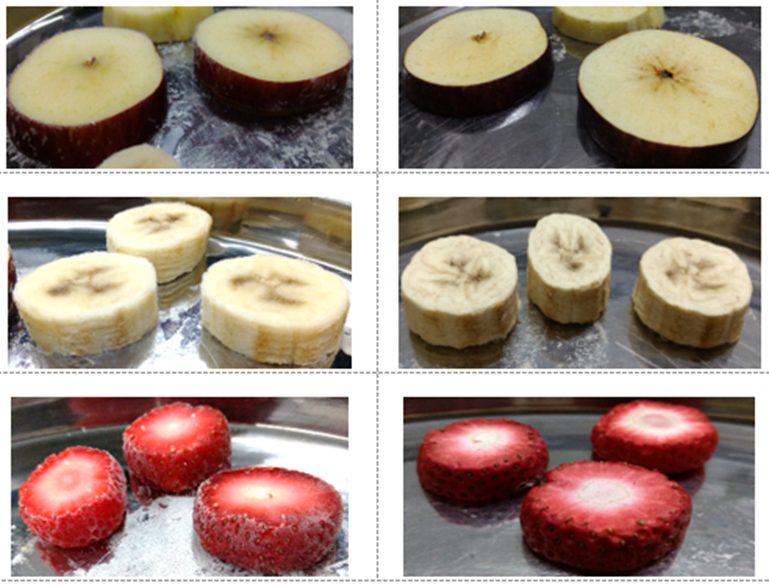
Figure Legend 7: Frozen and freeze-dried images of apple, banana, and strawberry: Left column for frozen fruits, right column for freeze-dried products.
A robust model was developed for sublimation drying of fruits, accounting for the increasing dried layer to predict remaining ice content. The symmetrical mass transfer model, fitted to experimental data for apple, banana, and strawberry, accurately represented observed behavior. Dried layer permeabilities (b) ranged from 2.3 to 5.4 ×10-9 kg water (m Pa s)-1. However, the relevant kinetic parameter was a combination of permeability and the relative mass of sublimed ice, whose order was congruent with experimental sublimation rates. The model innovatively used the unfrozen water fraction as the primary-secondary period limit. A falling sublimation rate, predicted for all three fruits, was attributed to the increasing dried layer thickness.
Secondary drying was modeled using the analytical solution of the diffusion equation. Accurate predictions for this low moisture content period yielded effective diffusion coefficients in high vacuum of 1.6 to 2.9 ×10-9 m2 s-1. These values are significantly higher than those reported for convective drying at atmospheric pressure, which suggest the creation of a porous structure. The order of D for the three fruits corresponded to their desorption rates and aligned with the order of permeabilities during the sublimation period, as both parameters relate to vapor migration through the porous structure.
Overall, this two-model approach for simulating fruit freeze-drying is accurate, well-founded, and computationally efficient, making it suitable for interactive freeze-dryer design and even as an automatic control algorithm.
We would like to express our sincere gratitude to the National Council of Scientific and Technical Research (CONICET) and the National University of La Plata for their generous financial support for this research project. Their contribution has been fundamental in carrying out this research and achieving the proposed objectives.
The authors declare that they have no conflict of interest, scientific or economic, related to this research.
Clearly Auctoresonline and particularly Psychology and Mental Health Care Journal is dedicated to improving health care services for individuals and populations. The editorial boards' ability to efficiently recognize and share the global importance of health literacy with a variety of stakeholders. Auctoresonline publishing platform can be used to facilitate of optimal client-based services and should be added to health care professionals' repertoire of evidence-based health care resources.

Journal of Clinical Cardiology and Cardiovascular Intervention The submission and review process was adequate. However I think that the publication total value should have been enlightened in early fases. Thank you for all.

Journal of Women Health Care and Issues By the present mail, I want to say thank to you and tour colleagues for facilitating my published article. Specially thank you for the peer review process, support from the editorial office. I appreciate positively the quality of your journal.
Journal of Clinical Research and Reports I would be very delighted to submit my testimonial regarding the reviewer board and the editorial office. The reviewer board were accurate and helpful regarding any modifications for my manuscript. And the editorial office were very helpful and supportive in contacting and monitoring with any update and offering help. It was my pleasure to contribute with your promising Journal and I am looking forward for more collaboration.

We would like to thank the Journal of Thoracic Disease and Cardiothoracic Surgery because of the services they provided us for our articles. The peer-review process was done in a very excellent time manner, and the opinions of the reviewers helped us to improve our manuscript further. The editorial office had an outstanding correspondence with us and guided us in many ways. During a hard time of the pandemic that is affecting every one of us tremendously, the editorial office helped us make everything easier for publishing scientific work. Hope for a more scientific relationship with your Journal.

The peer-review process which consisted high quality queries on the paper. I did answer six reviewers’ questions and comments before the paper was accepted. The support from the editorial office is excellent.

Journal of Neuroscience and Neurological Surgery. I had the experience of publishing a research article recently. The whole process was simple from submission to publication. The reviewers made specific and valuable recommendations and corrections that improved the quality of my publication. I strongly recommend this Journal.

Dr. Katarzyna Byczkowska My testimonial covering: "The peer review process is quick and effective. The support from the editorial office is very professional and friendly. Quality of the Clinical Cardiology and Cardiovascular Interventions is scientific and publishes ground-breaking research on cardiology that is useful for other professionals in the field.

Thank you most sincerely, with regard to the support you have given in relation to the reviewing process and the processing of my article entitled "Large Cell Neuroendocrine Carcinoma of The Prostate Gland: A Review and Update" for publication in your esteemed Journal, Journal of Cancer Research and Cellular Therapeutics". The editorial team has been very supportive.

Testimony of Journal of Clinical Otorhinolaryngology: work with your Reviews has been a educational and constructive experience. The editorial office were very helpful and supportive. It was a pleasure to contribute to your Journal.

Dr. Bernard Terkimbi Utoo, I am happy to publish my scientific work in Journal of Women Health Care and Issues (JWHCI). The manuscript submission was seamless and peer review process was top notch. I was amazed that 4 reviewers worked on the manuscript which made it a highly technical, standard and excellent quality paper. I appreciate the format and consideration for the APC as well as the speed of publication. It is my pleasure to continue with this scientific relationship with the esteem JWHCI.

This is an acknowledgment for peer reviewers, editorial board of Journal of Clinical Research and Reports. They show a lot of consideration for us as publishers for our research article “Evaluation of the different factors associated with side effects of COVID-19 vaccination on medical students, Mutah university, Al-Karak, Jordan”, in a very professional and easy way. This journal is one of outstanding medical journal.
Dear Hao Jiang, to Journal of Nutrition and Food Processing We greatly appreciate the efficient, professional and rapid processing of our paper by your team. If there is anything else we should do, please do not hesitate to let us know. On behalf of my co-authors, we would like to express our great appreciation to editor and reviewers.

As an author who has recently published in the journal "Brain and Neurological Disorders". I am delighted to provide a testimonial on the peer review process, editorial office support, and the overall quality of the journal. The peer review process at Brain and Neurological Disorders is rigorous and meticulous, ensuring that only high-quality, evidence-based research is published. The reviewers are experts in their fields, and their comments and suggestions were constructive and helped improve the quality of my manuscript. The review process was timely and efficient, with clear communication from the editorial office at each stage. The support from the editorial office was exceptional throughout the entire process. The editorial staff was responsive, professional, and always willing to help. They provided valuable guidance on formatting, structure, and ethical considerations, making the submission process seamless. Moreover, they kept me informed about the status of my manuscript and provided timely updates, which made the process less stressful. The journal Brain and Neurological Disorders is of the highest quality, with a strong focus on publishing cutting-edge research in the field of neurology. The articles published in this journal are well-researched, rigorously peer-reviewed, and written by experts in the field. The journal maintains high standards, ensuring that readers are provided with the most up-to-date and reliable information on brain and neurological disorders. In conclusion, I had a wonderful experience publishing in Brain and Neurological Disorders. The peer review process was thorough, the editorial office provided exceptional support, and the journal's quality is second to none. I would highly recommend this journal to any researcher working in the field of neurology and brain disorders.

Dear Agrippa Hilda, Journal of Neuroscience and Neurological Surgery, Editorial Coordinator, I trust this message finds you well. I want to extend my appreciation for considering my article for publication in your esteemed journal. I am pleased to provide a testimonial regarding the peer review process and the support received from your editorial office. The peer review process for my paper was carried out in a highly professional and thorough manner. The feedback and comments provided by the authors were constructive and very useful in improving the quality of the manuscript. This rigorous assessment process undoubtedly contributes to the high standards maintained by your journal.

International Journal of Clinical Case Reports and Reviews. I strongly recommend to consider submitting your work to this high-quality journal. The support and availability of the Editorial staff is outstanding and the review process was both efficient and rigorous.

Thank you very much for publishing my Research Article titled “Comparing Treatment Outcome Of Allergic Rhinitis Patients After Using Fluticasone Nasal Spray And Nasal Douching" in the Journal of Clinical Otorhinolaryngology. As Medical Professionals we are immensely benefited from study of various informative Articles and Papers published in this high quality Journal. I look forward to enriching my knowledge by regular study of the Journal and contribute my future work in the field of ENT through the Journal for use by the medical fraternity. The support from the Editorial office was excellent and very prompt. I also welcome the comments received from the readers of my Research Article.

Dear Erica Kelsey, Editorial Coordinator of Cancer Research and Cellular Therapeutics Our team is very satisfied with the processing of our paper by your journal. That was fast, efficient, rigorous, but without unnecessary complications. We appreciated the very short time between the submission of the paper and its publication on line on your site.

I am very glad to say that the peer review process is very successful and fast and support from the Editorial Office. Therefore, I would like to continue our scientific relationship for a long time. And I especially thank you for your kindly attention towards my article. Have a good day!

"We recently published an article entitled “Influence of beta-Cyclodextrins upon the Degradation of Carbofuran Derivatives under Alkaline Conditions" in the Journal of “Pesticides and Biofertilizers” to show that the cyclodextrins protect the carbamates increasing their half-life time in the presence of basic conditions This will be very helpful to understand carbofuran behaviour in the analytical, agro-environmental and food areas. We greatly appreciated the interaction with the editor and the editorial team; we were particularly well accompanied during the course of the revision process, since all various steps towards publication were short and without delay".

I would like to express my gratitude towards you process of article review and submission. I found this to be very fair and expedient. Your follow up has been excellent. I have many publications in national and international journal and your process has been one of the best so far. Keep up the great work.

We are grateful for this opportunity to provide a glowing recommendation to the Journal of Psychiatry and Psychotherapy. We found that the editorial team were very supportive, helpful, kept us abreast of timelines and over all very professional in nature. The peer review process was rigorous, efficient and constructive that really enhanced our article submission. The experience with this journal remains one of our best ever and we look forward to providing future submissions in the near future.

I am very pleased to serve as EBM of the journal, I hope many years of my experience in stem cells can help the journal from one way or another. As we know, stem cells hold great potential for regenerative medicine, which are mostly used to promote the repair response of diseased, dysfunctional or injured tissue using stem cells or their derivatives. I think Stem Cell Research and Therapeutics International is a great platform to publish and share the understanding towards the biology and translational or clinical application of stem cells.

I would like to give my testimony in the support I have got by the peer review process and to support the editorial office where they were of asset to support young author like me to be encouraged to publish their work in your respected journal and globalize and share knowledge across the globe. I really give my great gratitude to your journal and the peer review including the editorial office.

I am delighted to publish our manuscript entitled "A Perspective on Cocaine Induced Stroke - Its Mechanisms and Management" in the Journal of Neuroscience and Neurological Surgery. The peer review process, support from the editorial office, and quality of the journal are excellent. The manuscripts published are of high quality and of excellent scientific value. I recommend this journal very much to colleagues.

Dr.Tania Muñoz, My experience as researcher and author of a review article in The Journal Clinical Cardiology and Interventions has been very enriching and stimulating. The editorial team is excellent, performs its work with absolute responsibility and delivery. They are proactive, dynamic and receptive to all proposals. Supporting at all times the vast universe of authors who choose them as an option for publication. The team of review specialists, members of the editorial board, are brilliant professionals, with remarkable performance in medical research and scientific methodology. Together they form a frontline team that consolidates the JCCI as a magnificent option for the publication and review of high-level medical articles and broad collective interest. I am honored to be able to share my review article and open to receive all your comments.

“The peer review process of JPMHC is quick and effective. Authors are benefited by good and professional reviewers with huge experience in the field of psychology and mental health. The support from the editorial office is very professional. People to contact to are friendly and happy to help and assist any query authors might have. Quality of the Journal is scientific and publishes ground-breaking research on mental health that is useful for other professionals in the field”.

Dear editorial department: On behalf of our team, I hereby certify the reliability and superiority of the International Journal of Clinical Case Reports and Reviews in the peer review process, editorial support, and journal quality. Firstly, the peer review process of the International Journal of Clinical Case Reports and Reviews is rigorous, fair, transparent, fast, and of high quality. The editorial department invites experts from relevant fields as anonymous reviewers to review all submitted manuscripts. These experts have rich academic backgrounds and experience, and can accurately evaluate the academic quality, originality, and suitability of manuscripts. The editorial department is committed to ensuring the rigor of the peer review process, while also making every effort to ensure a fast review cycle to meet the needs of authors and the academic community. Secondly, the editorial team of the International Journal of Clinical Case Reports and Reviews is composed of a group of senior scholars and professionals with rich experience and professional knowledge in related fields. The editorial department is committed to assisting authors in improving their manuscripts, ensuring their academic accuracy, clarity, and completeness. Editors actively collaborate with authors, providing useful suggestions and feedback to promote the improvement and development of the manuscript. We believe that the support of the editorial department is one of the key factors in ensuring the quality of the journal. Finally, the International Journal of Clinical Case Reports and Reviews is renowned for its high- quality articles and strict academic standards. The editorial department is committed to publishing innovative and academically valuable research results to promote the development and progress of related fields. The International Journal of Clinical Case Reports and Reviews is reasonably priced and ensures excellent service and quality ratio, allowing authors to obtain high-level academic publishing opportunities in an affordable manner. I hereby solemnly declare that the International Journal of Clinical Case Reports and Reviews has a high level of credibility and superiority in terms of peer review process, editorial support, reasonable fees, and journal quality. Sincerely, Rui Tao.

Clinical Cardiology and Cardiovascular Interventions I testity the covering of the peer review process, support from the editorial office, and quality of the journal.

Clinical Cardiology and Cardiovascular Interventions, we deeply appreciate the interest shown in our work and its publication. It has been a true pleasure to collaborate with you. The peer review process, as well as the support provided by the editorial office, have been exceptional, and the quality of the journal is very high, which was a determining factor in our decision to publish with you.
The peer reviewers process is quick and effective, the supports from editorial office is excellent, the quality of journal is high. I would like to collabroate with Internatioanl journal of Clinical Case Reports and Reviews journal clinically in the future time.

Clinical Cardiology and Cardiovascular Interventions, I would like to express my sincerest gratitude for the trust placed in our team for the publication in your journal. It has been a true pleasure to collaborate with you on this project. I am pleased to inform you that both the peer review process and the attention from the editorial coordination have been excellent. Your team has worked with dedication and professionalism to ensure that your publication meets the highest standards of quality. We are confident that this collaboration will result in mutual success, and we are eager to see the fruits of this shared effort.

Dear Dr. Jessica Magne, Editorial Coordinator 0f Clinical Cardiology and Cardiovascular Interventions, I hope this message finds you well. I want to express my utmost gratitude for your excellent work and for the dedication and speed in the publication process of my article titled "Navigating Innovation: Qualitative Insights on Using Technology for Health Education in Acute Coronary Syndrome Patients." I am very satisfied with the peer review process, the support from the editorial office, and the quality of the journal. I hope we can maintain our scientific relationship in the long term.
Dear Monica Gissare, - Editorial Coordinator of Nutrition and Food Processing. ¨My testimony with you is truly professional, with a positive response regarding the follow-up of the article and its review, you took into account my qualities and the importance of the topic¨.

Dear Dr. Jessica Magne, Editorial Coordinator 0f Clinical Cardiology and Cardiovascular Interventions, The review process for the article “The Handling of Anti-aggregants and Anticoagulants in the Oncologic Heart Patient Submitted to Surgery” was extremely rigorous and detailed. From the initial submission to the final acceptance, the editorial team at the “Journal of Clinical Cardiology and Cardiovascular Interventions” demonstrated a high level of professionalism and dedication. The reviewers provided constructive and detailed feedback, which was essential for improving the quality of our work. Communication was always clear and efficient, ensuring that all our questions were promptly addressed. The quality of the “Journal of Clinical Cardiology and Cardiovascular Interventions” is undeniable. It is a peer-reviewed, open-access publication dedicated exclusively to disseminating high-quality research in the field of clinical cardiology and cardiovascular interventions. The journal's impact factor is currently under evaluation, and it is indexed in reputable databases, which further reinforces its credibility and relevance in the scientific field. I highly recommend this journal to researchers looking for a reputable platform to publish their studies.
Dear Editorial Coordinator of the Journal of Nutrition and Food Processing! "I would like to thank the Journal of Nutrition and Food Processing for including and publishing my article. The peer review process was very quick, movement and precise. The Editorial Board has done an extremely conscientious job with much help, valuable comments and advices. I find the journal very valuable from a professional point of view, thank you very much for allowing me to be part of it and I would like to participate in the future!”

Dealing with The Journal of Neurology and Neurological Surgery was very smooth and comprehensive. The office staff took time to address my needs and the response from editors and the office was prompt and fair. I certainly hope to publish with this journal again.Their professionalism is apparent and more than satisfactory. Susan Weiner

My Testimonial Covering as fellowing: Lin-Show Chin. The peer reviewers process is quick and effective, the supports from editorial office is excellent, the quality of journal is high. I would like to collabroate with Internatioanl journal of Clinical Case Reports and Reviews.

My experience publishing in Psychology and Mental Health Care was exceptional. The peer review process was rigorous and constructive, with reviewers providing valuable insights that helped enhance the quality of our work. The editorial team was highly supportive and responsive, making the submission process smooth and efficient. The journal's commitment to high standards and academic rigor makes it a respected platform for quality research. I am grateful for the opportunity to publish in such a reputable journal.
My experience publishing in International Journal of Clinical Case Reports and Reviews was exceptional. I Come forth to Provide a Testimonial Covering the Peer Review Process and the editorial office for the Professional and Impartial Evaluation of the Manuscript.

I would like to offer my testimony in the support. I have received through the peer review process and support the editorial office where they are to support young authors like me, encourage them to publish their work in your esteemed journals, and globalize and share knowledge globally. I really appreciate your journal, peer review, and editorial office.
Dear Agrippa Hilda- Editorial Coordinator of Journal of Neuroscience and Neurological Surgery, "The peer review process was very quick and of high quality, which can also be seen in the articles in the journal. The collaboration with the editorial office was very good."

I would like to express my sincere gratitude for the support and efficiency provided by the editorial office throughout the publication process of my article, “Delayed Vulvar Metastases from Rectal Carcinoma: A Case Report.” I greatly appreciate the assistance and guidance I received from your team, which made the entire process smooth and efficient. The peer review process was thorough and constructive, contributing to the overall quality of the final article. I am very grateful for the high level of professionalism and commitment shown by the editorial staff, and I look forward to maintaining a long-term collaboration with the International Journal of Clinical Case Reports and Reviews.
To Dear Erin Aust, I would like to express my heartfelt appreciation for the opportunity to have my work published in this esteemed journal. The entire publication process was smooth and well-organized, and I am extremely satisfied with the final result. The Editorial Team demonstrated the utmost professionalism, providing prompt and insightful feedback throughout the review process. Their clear communication and constructive suggestions were invaluable in enhancing my manuscript, and their meticulous attention to detail and dedication to quality are truly commendable. Additionally, the support from the Editorial Office was exceptional. From the initial submission to the final publication, I was guided through every step of the process with great care and professionalism. The team's responsiveness and assistance made the entire experience both easy and stress-free. I am also deeply impressed by the quality and reputation of the journal. It is an honor to have my research featured in such a respected publication, and I am confident that it will make a meaningful contribution to the field.

"I am grateful for the opportunity of contributing to [International Journal of Clinical Case Reports and Reviews] and for the rigorous review process that enhances the quality of research published in your esteemed journal. I sincerely appreciate the time and effort of your team who have dedicatedly helped me in improvising changes and modifying my manuscript. The insightful comments and constructive feedback provided have been invaluable in refining and strengthening my work".

I thank the ‘Journal of Clinical Research and Reports’ for accepting this article for publication. This is a rigorously peer reviewed journal which is on all major global scientific data bases. I note the review process was prompt, thorough and professionally critical. It gave us an insight into a number of important scientific/statistical issues. The review prompted us to review the relevant literature again and look at the limitations of the study. The peer reviewers were open, clear in the instructions and the editorial team was very prompt in their communication. This journal certainly publishes quality research articles. I would recommend the journal for any future publications.

Dear Jessica Magne, with gratitude for the joint work. Fast process of receiving and processing the submitted scientific materials in “Clinical Cardiology and Cardiovascular Interventions”. High level of competence of the editors with clear and correct recommendations and ideas for enriching the article.

We found the peer review process quick and positive in its input. The support from the editorial officer has been very agile, always with the intention of improving the article and taking into account our subsequent corrections.

My article, titled 'No Way Out of the Smartphone Epidemic Without Considering the Insights of Brain Research,' has been republished in the International Journal of Clinical Case Reports and Reviews. The review process was seamless and professional, with the editors being both friendly and supportive. I am deeply grateful for their efforts.
To Dear Erin Aust – Editorial Coordinator of Journal of General Medicine and Clinical Practice! I declare that I am absolutely satisfied with your work carried out with great competence in following the manuscript during the various stages from its receipt, during the revision process to the final acceptance for publication. Thank Prof. Elvira Farina

Dear Jessica, and the super professional team of the ‘Clinical Cardiology and Cardiovascular Interventions’ I am sincerely grateful to the coordinated work of the journal team for the no problem with the submission of my manuscript: “Cardiometabolic Disorders in A Pregnant Woman with Severe Preeclampsia on the Background of Morbid Obesity (Case Report).” The review process by 5 experts was fast, and the comments were professional, which made it more specific and academic, and the process of publication and presentation of the article was excellent. I recommend that my colleagues publish articles in this journal, and I am interested in further scientific cooperation. Sincerely and best wishes, Dr. Oleg Golyanovskiy.

Dear Ashley Rosa, Editorial Coordinator of the journal - Psychology and Mental Health Care. " The process of obtaining publication of my article in the Psychology and Mental Health Journal was positive in all areas. The peer review process resulted in a number of valuable comments, the editorial process was collaborative and timely, and the quality of this journal has been quickly noticed, resulting in alternative journals contacting me to publish with them." Warm regards, Susan Anne Smith, PhD. Australian Breastfeeding Association.

Dear Jessica Magne, Editorial Coordinator, Clinical Cardiology and Cardiovascular Interventions, Auctores Publishing LLC. I appreciate the journal (JCCI) editorial office support, the entire team leads were always ready to help, not only on technical front but also on thorough process. Also, I should thank dear reviewers’ attention to detail and creative approach to teach me and bring new insights by their comments. Surely, more discussions and introduction of other hemodynamic devices would provide better prevention and management of shock states. Your efforts and dedication in presenting educational materials in this journal are commendable. Best wishes from, Farahnaz Fallahian.
Dear Maria Emerson, Editorial Coordinator, International Journal of Clinical Case Reports and Reviews, Auctores Publishing LLC. I am delighted to have published our manuscript, "Acute Colonic Pseudo-Obstruction (ACPO): A rare but serious complication following caesarean section." I want to thank the editorial team, especially Maria Emerson, for their prompt review of the manuscript, quick responses to queries, and overall support. Yours sincerely Dr. Victor Olagundoye.

Dear Ashley Rosa, Editorial Coordinator, International Journal of Clinical Case Reports and Reviews. Many thanks for publishing this manuscript after I lost confidence the editors were most helpful, more than other journals Best wishes from, Susan Anne Smith, PhD. Australian Breastfeeding Association.

Dear Agrippa Hilda, Editorial Coordinator, Journal of Neuroscience and Neurological Surgery. The entire process including article submission, review, revision, and publication was extremely easy. The journal editor was prompt and helpful, and the reviewers contributed to the quality of the paper. Thank you so much! Eric Nussbaum, MD
Dr Hala Al Shaikh This is to acknowledge that the peer review process for the article ’ A Novel Gnrh1 Gene Mutation in Four Omani Male Siblings, Presentation and Management ’ sent to the International Journal of Clinical Case Reports and Reviews was quick and smooth. The editorial office was prompt with easy communication.

Dear Erin Aust, Editorial Coordinator, Journal of General Medicine and Clinical Practice. We are pleased to share our experience with the “Journal of General Medicine and Clinical Practice”, following the successful publication of our article. The peer review process was thorough and constructive, helping to improve the clarity and quality of the manuscript. We are especially thankful to Ms. Erin Aust, the Editorial Coordinator, for her prompt communication and continuous support throughout the process. Her professionalism ensured a smooth and efficient publication experience. The journal upholds high editorial standards, and we highly recommend it to fellow researchers seeking a credible platform for their work. Best wishes By, Dr. Rakhi Mishra.

Dear Jessica Magne, Editorial Coordinator, Clinical Cardiology and Cardiovascular Interventions, Auctores Publishing LLC. The peer review process of the journal of Clinical Cardiology and Cardiovascular Interventions was excellent and fast, as was the support of the editorial office and the quality of the journal. Kind regards Walter F. Riesen Prof. Dr. Dr. h.c. Walter F. Riesen.

Dear Ashley Rosa, Editorial Coordinator, International Journal of Clinical Case Reports and Reviews, Auctores Publishing LLC. Thank you for publishing our article, Exploring Clozapine's Efficacy in Managing Aggression: A Multiple Single-Case Study in Forensic Psychiatry in the international journal of clinical case reports and reviews. We found the peer review process very professional and efficient. The comments were constructive, and the whole process was efficient. On behalf of the co-authors, I would like to thank you for publishing this article. With regards, Dr. Jelle R. Lettinga.

Dear Clarissa Eric, Editorial Coordinator, Journal of Clinical Case Reports and Studies, I would like to express my deep admiration for the exceptional professionalism demonstrated by your journal. I am thoroughly impressed by the speed of the editorial process, the substantive and insightful reviews, and the meticulous preparation of the manuscript for publication. Additionally, I greatly appreciate the courteous and immediate responses from your editorial office to all my inquiries. Best Regards, Dariusz Ziora

Dear Chrystine Mejia, Editorial Coordinator, Journal of Neurodegeneration and Neurorehabilitation, Auctores Publishing LLC, We would like to thank the editorial team for the smooth and high-quality communication leading up to the publication of our article in the Journal of Neurodegeneration and Neurorehabilitation. The reviewers have extensive knowledge in the field, and their relevant questions helped to add value to our publication. Kind regards, Dr. Ravi Shrivastava.

Dear Clarissa Eric, Editorial Coordinator, Journal of Clinical Case Reports and Studies, Auctores Publishing LLC, USA Office: +1-(302)-520-2644. I would like to express my sincere appreciation for the efficient and professional handling of my case report by the ‘Journal of Clinical Case Reports and Studies’. The peer review process was not only fast but also highly constructive—the reviewers’ comments were clear, relevant, and greatly helped me improve the quality and clarity of my manuscript. I also received excellent support from the editorial office throughout the process. Communication was smooth and timely, and I felt well guided at every stage, from submission to publication. The overall quality and rigor of the journal are truly commendable. I am pleased to have published my work with Journal of Clinical Case Reports and Studies, and I look forward to future opportunities for collaboration. Sincerely, Aline Tollet, UCLouvain.

Dear Ms. Mayra Duenas, Editorial Coordinator, International Journal of Clinical Case Reports and Reviews. “The International Journal of Clinical Case Reports and Reviews represented the “ideal house” to share with the research community a first experience with the use of the Simeox device for speech rehabilitation. High scientific reputation and attractive website communication were first determinants for the selection of this Journal, and the following submission process exceeded expectations: fast but highly professional peer review, great support by the editorial office, elegant graphic layout. Exactly what a dynamic research team - also composed by allied professionals - needs!" From, Chiara Beccaluva, PT - Italy.

Dear Maria Emerson, Editorial Coordinator, we have deeply appreciated the professionalism demonstrated by the International Journal of Clinical Case Reports and Reviews. The reviewers have extensive knowledge of our field and have been very efficient and fast in supporting the process. I am really looking forward to further collaboration. Thanks. Best regards, Dr. Claudio Ligresti